Where's the Math in MathMol?

Numerous connections are found between mathematics and molecular modeling. Here are just a few!

275K Movie

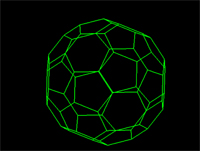
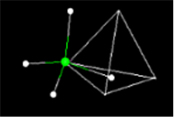
Many molecular structures have the same shape of familiar space figures. Take another look at the fullerene molecule shown above. The molecule has the same shape as an icosohedron. Notice that the faces are composed of hexagons and pentagons. The picture above shows another familiar space figure, that of the tetrahedron. Click on the picture and watch how the methane is superimposed onto the tetrahedron.

5K Images

Angles are formed by the intersection of two rays (or line segments). The figure on the left shows a parallelogram with one base angle equal to 112 degrees. We measure angles formed by molecular bonds in a similar way, using three consecutive atoms to represent three points on a plane. The figure on the right shows the bond angle formed by 3 consecutive carbon atoms in a benzene ring.

5K Images

Dihedral angles are formed by the intersection of two planes in space. On the left, two intersecting planes form a dihedral angle of 90 degrees. On the right, the dihedral angle shown for the hexane molecule is 59.4 degrees.

406K Movie

A line is a set of points described by an equation which may be written in the form y=mx+b where the constant m is the slope of the line and b represents its y intercept. As x varies y varies. In the figure above, as the temperature (T) of the system varies, the kinetic energy (E) of the molecule varies. The line describes the equation E=kT where k is the slope of the line.

1066K Movie

The set of points which satisfy the equation y=1/x is a hyperbola. As x varies y varies inversely. As x becomes smaller y becomes larger. The movie above shows two particles of opposite charge attracted to each other. The relationship between coulombic energy and distance is an inverse relationship. The coulombic energy between the two particles varies with -1/r, where r is the distance separating their center of masses. In the movie notice that as r gets smaller, the potential energy becomes more negative.

753K Movie

The movie above shows that the torsion bond energy of a molecule can be modeled by a periodic function.

Image 5K

Many geometric figures display symmetry. The equilateral triangle on the left displays rotational symmetry. Rotating the figure about about a point formed by the intersection of the three angle bisectors (the incenter of the triangle) will give the same appearance. The ammonia molecule on the right also shows symmetry. It has a 3-fold axis of symmetry. Rotating the molecule 120 degrees about its axis will give the same appearance.